Class 9 NCERT Solutions Physics Chapter 10 - Work and Energy

When a force F acts on an object to displace it through a distance s in its direction, then the work done W on the object by the force is given by:

Work done = Force × Displacement

W = F × s

Given:

Force, F = 7 N

Displacement, s = 8 m  

Therefore, work done is,

W = F × s

W = 7 N × 8 m

   = 56 Nm

W = 56 J

Work is done whenever the given conditions are satisfied:

(i) A force acts on a body.

(ii) There is a displacement of the body.

When a force F displaces a body through a distance s in the direction of the applied force, then the work done W on the body is given by the expression:

Work done = Force × Displacement

W = F × s   

1 J is the amount of work done by a force of 1 N on an object, to displace it through a distance of 1 m in its own direction. 

Work done by the bullocks is given by the expression:

Work done = Force × Displacement

W = F ×  s

Where,

Applied force, F = 140 N

Displacement, s = 15 m

W = 140  × 15 = 2100 J   

Hence, 2100 J of work is done in ploughing the length of the field.

Kinetic energy is the energy possessed by a body by the virtue of its motion. Every moving body possesses kinetic energy. In fact, kinetic energy of a body moving with a certain velocity is equal to the work done to make it acquire that velocity. 

If an object of mass m is moving with a velocity v, then its kinetic energy E_k is given by the expression,

Expression for kinetic energy is

m = Mass of the object

v = Velocity of the object = 5 ms^-1

Given that kinetic energy, E_k= 25 J

(i) If the velocity of an object is doubled, then its kinetic energy becomes 4 times the original value, because it is proportional to the square of the velocity. Hence, kinetic energy = 25 × 4 = 100 J.  

(ii) If velocity is increased three times, then its kinetic energy becomes 9 times the original value, because it is proportional to the square of the velocity. Hence, kinetic energy = 25  × 9 = 225 J.

Power is the rate of doing work or the rate of transfer of energy. If W is the amount of work done in time t, then power is given by the expression,

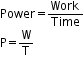
It is expressed in watt (W).

A body is said to have power of 1 watt if it does work at the rate of 1 joule per second, i.e.,

Power is given by the expression,

Work done = Energy consumed by the lamp = 1000 J

Time = 10 s

Average power is obtained by dividing the total amount of work done by the total time taken to do the work.

The concept of average power is used when the power of an agent varies with time i.e. it does work at different rates during different intervals of time.

Work is done whenever the given conditions are satisfied:

(i) A force acts on a body.

(ii) There is a displacement of the body.

 (a) While swimming, Suma applies a force to push the water backwards. Therefore, Suma swims in the forward direction caused by the forward reaction of water. Here, the force causes a displacement. Hence, work is done by Seema while swimming.

(b) While carrying a load, the donkey has to apply a force in the upward direction. But, displacement of the load is in the forward direction. Since, displacement is perpendicular to force, the work done is zero.

(c) A wind mill works against the gravitational force to lift water. Hence, work is done by the wind mill in lifting water from the well.

(d) In this case, there is no displacement of the leaves of the plant. Therefore, the work done is zero.

(e) An engine applies force to pull the train. This allows the train to move in the direction of force. Therefore, there is a displacement in the train in the same direction. Hence, work is done by the engine on the train.

(f) Food grains do not move in the presence of solar energy. Hence, the work done is zero during the process of food grains getting dried in the Sun.

(g) Wind energy applies a force on the sailboat to push it in the forward direction. Therefore, there is a displacement in the boat in the direction of force. Hence, work is done by wind on the boat.

Work done by the force of gravity on an object depends on vertical displacement. Vertical displacement is given by the difference between the initial and final heights of the object, which is zero.

Work done by gravity is given by the expression,

W = mgh

where,

h = Vertical displacement = 0

W = mg × 0 = 0 J

Therefore, the work done by gravity on the given object is zero.

When a bulb is connected to a battery, then the chemical energy of the battery is transferred into electrical energy. When the bulb receives this electrical energy, then it converts it into light and heat energy. Hence, the transformation of energy in the given situation can be shown as:

Mass of the body, m = 20 kg

Initial velocity, u = 5 m/s

Final velocity, v = 2 m/s

Initial kinetic energy of the body,

Final kinetic energy of the body,

Work done by the force = Change in kinetic energy

                               = Final kinetic energy - Initial kinetic energy

                               = E_kf - E_ki

                               = 40 J - 250 J

                               = -210 J

Work done is negative because force is applied in the direction opposite to that of displacement.

Work done on the object by the gravitational force is zero. This is because the force of gravity and displacement of the body are at right angles to each other.

No. The process does not violate the law of conservation of energy. This is because when the body falls from a height, then its potential energy changes into kinetic energy progressively. A decrease in the potential energy is equal to an increase in the kinetic energy of the body. During the process, total mechanical energy of the body remains conserved. Therefore, the law of conservation of energy holds good.

The muscular energy of the cyclist is converted into rotational kinetic energy of the pedals of the bicycle which is transferred to its wheels. Due to this, the bicycle wheels move forward. When the bicycle moves, then the bicycle as well as the person riding the bicycle, both have kinetic energy.

When we push a huge rock and fail to move it, the energy spent by us gets stored in the rock as potential energy of configuration which results in its deformation. However, this deformation in the rock is so small that it cannot be observed by us.

1 unit of energy is equal to 1 kilowatt hour (kWh).

1 unit = 1 kWh

1 kWh = 3.6 × 10⁶ J

Therefore, 250 units of energy = 250  × 3.6 × 10⁶ = 9 × 10⁸ J

Gravitational potential energy is given by the expression,

W = m g h

where,

h = Height above the ground = 5 m

m = Mass of the object = 40 kg

g = Acceleration due to gravity = 9.8 ms^-2

W = 40 × 5  × 9.8 = 1960 J.

At half-way down, the potential energy of the object will be  = 980 J.

At this point, the object has an equal amount of potential and kinetic energy. This is due to the law of conservation of energy. Hence, half-way down, the kinetic energy of the object will also be 980 J.

When a satellite moves round the Earth, then at each point of its path, the direction of force of gravity on the satellite (along the radius) is perpendicular to the direction of its displacement (along the tangent). Hence, the work done on the satellite by the force of gravity is zero.

Yes. This is possible for an object undergoing uniform motion along a straight line.

We know, F = ma

If F = 0, then m x a = 0

But m cannot be zero, so a=0.

In such a case, the object is either at rest or moving with constant velocity (i.e. uniform motion along a straight line).

Therefore, when the object moves with constant velocity, there is a displacement of the object without any force acting on it.

When a person holds a bundle of hay over his head, then there is no displacement of the bundle at all. So, no work is done by him. However, the person gets tired due to the muscular fatigue experienced by him.

Energy consumed by an electric heater can be obtained with the help of the expression,

where,

Power rating of the heater, P = 1500 W = 1.5 kW

Time for which the heater has operated, t = 10 h

Work done = Energy consumed by the heater

Therefore, energy consumed = Power  × Time

                                         = 1.5 × 10 = 15 kWh

Hence, the energy consumed by the heater in 10 h is 15 kWh or 15 units.

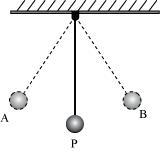
When the bob of the pendulum is drawn from its mean position P to either of its extreme positions (say B), it rises through a height and gains potential energy.

When it is released from position B and starts moving towards position P, its potential energy keeps on decreasing and its kinetic energy keeps on increasing.

When the bob reaches position P, its kinetic energy becomes maximum and potential energy becomes zero.

Now, when the bob starts moving to the other extreme position A, its kinetic energy goes on decreasing and its potential energy goes on increasing.

At position A, all the kinetic energy gets converted to potential energy.

Hence, we conclude that at the extreme positions A and B, all the energy of the bob is potential and at the mean position P, all the energy is kinetic. At all other intermediate positions, the energy of the bob is partly potential and partly kinetic. But the total energy at any instant remains constant.

The bob does not oscillate forever. It eventually comes to rest due to air resistance and the friction at the point of support. The law of conservation of energy is not violated in this case because the energy of the bob gets converted into heat energy and sound energy which go into the surroundings.

Kinetic energy of an object of mass m moving with a velocity v is given by the expression . To bring the object to rest, an equal amount of work i.e. is required to be done on the object.

Mass of car, m = 1500 kg

Velocity of car, v = 60 km/h =

Kinetic energy,

To stop the car, an amount of work equal to E_k is required to be done.

Hence, 20.8 × 10⁴ J of work is required to stop the car.  

Case I

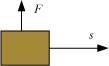
In this case, the direction of force acting on the block is perpendicular to the direction of displacement. Therefore, work done by force on the block will be zero.

Case II

In this case, the direction of force acting on the block and the direction of displacement is same. Therefore, work done by force on the block will be positive.

Case III

In this case, the direction of force acting on the block is opposite to the direction of displacement. Therefore, work done by force on the block will be negative.

Yes, acceleration in an object could be zero even when several forces are acting on it. This happens when all the forces cancel out each other i.e., the net force acting on the object is zero.

Power rating of each device, P = 500 W = 0.50 kW

Time for which each device runs, t = 10 h

Work done = Energy consumed by each device (E)

We know,

Energy consumed by each device= Power × Time

E = P x t

   = 0.50×10 = 5 kWh

Hence, the energy consumed by four devices of power 500 W each in 10 h will be

4 × 5 kWh = 20 kWh = 20 units

As the object hits the hard ground, its kinetic energy gets converted into

(i) heat energy (the object and the ground become slightly warm)

(ii) sound energy (sound is heard when the object hits the ground)

(iii) potential energy of configuration of the body and the ground (the object and the ground get deformed a little bit at the point of collision).